SIC POVMs and Zauner’s Conjecture – Open Quantum Problems

Cite this problem as Problem 23.

Problem

We will give three variants of the problem, each being stronger than its predecessor. The terminology of problems 1 and 2 is taken mainly from [1]. For problem 3 see [2] and [3].

Problem 1: SIC-POVMs

A set of $d^2$ normed vectors $\{|\phi_i>\}_i$ in a Hilbert space of dimension $d$ constitutes a set of <strong>equiangular lines</strong> if their mutual inner products $\left|<\phi_i|\phi_j>\right|^2$ are independent of the choice of $i \neq j$ . It can be shown [1] that

*the associated projection operators sum to a multiple of unity and thus induce a POVM (up to normalization) and that

*these operators are linearly independent and hence any quantum state can be reconstructed from the measurement statistics $p_i := tr\left(|\phi_i> <\phi_i| \rho \right)$ of the POVM.

A POVM that arises in this way is called symmetric informationally complete, or a SIC-POVM for short. The most general form of the problem is: decide if SIC-POVMs exists in any dimension $d$ .

Problem 2: covariant POVMs

For a given basis $\left\{|q>\right\}_{q=0\ldots d-1}$ of the Hilbert space, define the shift operator $X$ and clock operator $Z$ respectively by the relations $X |q> := |q+1>$ , $Z |q> := e^{i \frac {2 \pi} d q} |q>$ , where arithmetic is modulo $d$ . Further, define the Weyl operators

$w(p,q) = Z(p) X(q) \quad (*)$

for all $p, q \in {\mathbf{Z}}_d$ . We will refer to the group generated by (*) as the Heisenberg group. It is also known as the Weyl-Heisenberg group or Generalized Pauli group.

A vector $|\phi>$ is called a fiducial vector with respect to the Heisenberg group if the set

$\left\{w(p,q) \, |\phi> <\phi| \, w(p,q)^\ast \right\}_{p,q=0\ldots d-1}\quad (**)$

induces a SIC-POVM. Such a SIC-POVM is said to be group covariant. The definition makes sense for any group of order at least $d^2$ . However, we will focus on the Heisenberg group in what follows.

The problem: decide if group covariant SIC-POVMs exist in any dimension $d$ .

Problem 3: Zauner’s conjecture

The normalizer of the Heisenberg group within the unitaries $U(d)$ is called the Clifford group. There exists an element $z$ of the Clifford group which is defined via its action on the Weyl operators as $z \,w(p,q) z^\ast = w(q-p,-p).$ Zauner’s conjecture, as formulated in [3], runs: in any dimension $d$ , a fiducial vector can be found among the eigenvectors of $Z$ .

Background

Besides their mathematical appeal, SIC-POVMs have obvious applications to quantum state tomography. The symmetry condition assures that the possible measurement outcomes are in some sense maximally complementary.

Partial Results

In the context of quantum information, the problem seems to have been tackled first by Gerhard Zauner in his doctorial thesis [2] in 1999. To our knowledge, the results were neither published nor translated into English, which caused some confusion in the English literature, as to what Zauner had actually conjectured (Refer e.g. to the first vs. the second version of [3] on the arXiv server.). Zauner analyzed the spectrum of z
He listed analytical expressions for fiducial vectors in dimension 2, 3, 4, 5 and numerical expressions for d=6,7
He noted that for dimension 8 an analytic SIC-POVM is known, which is covariant under the action of the threefold tensor product of the two dimensional Heisenberg group.
Wide interest in the problem arose with the 2003 paper by Renes et. al. [1]. Building on concepts from frame theory, the authors reduced the task of numerically finding fiducial vectors to a non-convex global optimization problem. Using this method, they presented numerical fiducial vectors for all dimensions up to 45 and counted the number of distinct covariant SIC-POVMs up to dimension 7. The question of whether those vectors were eigenstates of a Clifford operation was left open (but see below). Further, four groups other than the Heisenberg group were numerically found to induce SIC-POVMs in the sense of (**). The authors showed that a SIC-POVM corresponds to a spherical 2-design (A finite set X of unit vectors is a t-design if the average of any t-th order polynomial over X is the same as the average of that polynomial over the entire unit sphere.). The same assertion was proven by Klappenecker and Rötteler in [4] and was apparently known to Zauner (see Remark 3 in [4]).
In [5] Grassl used a computer algebra system capable of symbolic calculations to prove Zauner’s conjecture for $d=6$ .
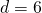He remarked that elements of the Clifford group map fiducial vectors onto fiducial vectors. Building on that observation, he could account for all 96 covariant SIC-POVMs that were reported to exist for d=6 in [1].
Appleby in [3] gave a detailed description of the Clifford group and extended it by allowing for anti-unitary operators. He verified that the numeric solutions of [1] were compatible with Zauner’s conjecture and analyzed their stability groups inside the Clifford group (A similar analysis can be performed using discrete Wigner functions, as will be reported in [6]. Appleby goes on to present analytical expressions for fiducial vectors in dimension 7 and 19 and specifies an infinite sequence of dimensions for which he conjectures that solutions can be found more easily.
Inspired by a construction that links finite geometries to MUBs, there have been some speculations by Wootters about whether SIC-POVMs can be linked to finite affine planes [7]. The same line of thought was pursued by Bengtsson and Ericsson in [8]. However, the existence of such a construction remains an open problem. The results by Grassl are of some relevance here, as it is known that affine planes of order 6 do not exist.

References

[1] J. M. Renes, R. Blume-Kohout, A. J. Scott, and C. M. Caves, Symmetric Informationally Complete Quantum Measurements, J. Math. Phys. 45, 2171 (2004) and http://xxx.lanl.gov/abs/quant-ph/0310075(2003).

[2] G. Zauner, Quantendesigns — Grundzüge einer nichtkommutativen Designtheorie, Doctorial thesis, University of Vienna, 1999 (available online at http://www.mat.univie.ac.at/~neum/papers/physpapers.html

[3] D. M. Appleby, SIC-POVMs and the Extended Clifford Group, http://xxx.lanl.gov/abs/quant-ph/0412001

[4] A. Klappenecker, and M. Rötteler, Mutually Unbiased Bases are Complex Projective 2-Designs, http://xxx.lanl.gov/abs/quant-ph/0502031

[5] M. Grassl, On SIC-POVMs and MUBs in dimension 6, http://xxx.lanl.gov/abs/quant-ph/0406175

[6] D. Gross, Diploma thesis, University of Potsdam, 2005

[7] W. K. Wootters, Quantum measurements and finite geometry, http://xxx.lanl.gov/abs/quant-ph/0406032

[8] I. Bengtsson and \AA. Ericsson, Mutually Unbiased Bases and The Complementarity Polytope, http://xxx.lanl.gov/abs/quant-ph/0410120