Cite this problem as Problem 11.
Problem
There are different notions of capacity for a noisy quantum communication channel, e. g. for classical, private classical or quantum communications. Continuity of these is an important property, but from a mathematical point of view it is not at all obvious, because very similar channels can differ a lot given many copies, and the capacity is operationally defined in terms of an asymptotic number of channel uses. Of course this is not a problem when a single-letter formula is available, so that one can reason about it directly, but it becomes quite a challenge, when only a multi-letter formula is available, or even non at all.
Background
From a practical point of view, we wish for continuity, because in real systems there is always some channel uncertainty. So if nearby channels had dramatically different capacities, the theory of quantum capacities would be of limited value. Listed below are definitions of different capacities
- Classical Capacity: A rate R is
 -classically-achievable if there is an
-classically-achievable if there is an 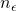 such that for all
such that for all 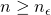 there is a classical code
there is a classical code 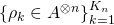 and a decoding operation
and a decoding operation 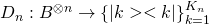 such that for all k,
such that for all k, 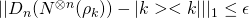 with log
with log 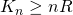 . A rate is classically-achievable if it is
. A rate is classically-achievable if it is  -classically-achievable for all
-classically-achievable for all 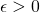 . The classical capacity of
. The classical capacity of 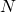 ,
, 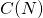 , is the supremum over classically-achievable rates.
, is the supremum over classically-achievable rates. - Quantum Capacity: A rate R is
 -achievable if there is an
-achievable if there is an 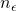 such that for all
such that for all 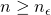 there is a quantum code,
there is a quantum code, 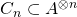 and a decoding operation
and a decoding operation 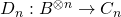 such that for all
such that for all 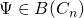 ,
,  and log dim
and log dim 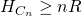 . A rate is achievable if it is
. A rate is achievable if it is  -achievable for all
-achievable for all 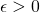 . The quantum capacity of
. The quantum capacity of 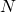 ,
, 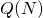 , is the supremum over achievable rates.
, is the supremum over achievable rates. - Private Capacity: The private capacity is the capacity of a channel for classical communication with the added requirement that an adversary with access to the environment of the channel is ignorant of the communication. More formally a rate R is
 -privately-achievable if there is an
-privately-achievable if there is an 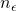 such that for all
such that for all 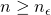 there is a classical code
there is a classical code 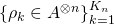 with log
with log 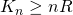 and a decoding operation
and a decoding operation 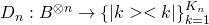 such that for all k,
such that for all k, 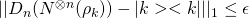 and
and 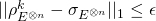 . Here
. Here 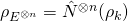 , where
, where  , with
, with 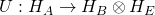 an isometric extension of
an isometric extension of 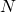 , and
, and 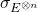 is a fixed state on
is a fixed state on 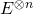 . A rate is privately-achievable if it is
. A rate is privately-achievable if it is  -privately-achievable for all
-privately-achievable for all 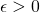 . The private capacity of
. The private capacity of 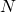 ,
, 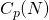 , is the supremum over privately-achievable rates.
, is the supremum over privately-achievable rates.
Partial Results
On the way to prove continuity of channel capacities, various intermediate results could be achieved. These contain results for capacities itself or quantities related to it, i. e. entropic quantities and entanglement measures.
- In [1] the continuity of the quantum channel capacity was assumed to upper bound the capacity of the quantum erasure channel, which was rigorously justified later in [2].
- In [3] it was shown, that the quantum channel capacity is lower semi-continuous.
- In [4] the continuity of the Holevo information was considered (which is connected to the classical capacity through regularization) and it was shown that it is continuous for finite dimensional outputs and lower semi-continuous in general.
- In [5] a tight bound on the variation of von Neumann entropy of finite dimensional states was found.
- In [6] the latter was used to study the entanglement of formation.
- In [7] the continuity of the relative entropy of entanglement was proven.
- In [8] asymptotic entanglement measures were proven to be continuous in any open set of distallable states.
- In [9] this continuity result was generalized to conditional entropy, which was used in [10] to prove the continuity of squashed entanglement.
Solution
The problem was essentially solved in [11]. There it was shown that the classical capacity, the quantum capacity and the private classical capacity are continuous (with respect to the diamond norm), where the variation on arguments ![]() apart is bounded by a simple function of
apart is bounded by a simple function of ![]() and the channel’s output dimension. Furthermore for quantum capacities in the presence of free backward or two-way classical communication, continuity was proven on the interior of the set of non-zero capacity channels.
and the channel’s output dimension. Furthermore for quantum capacities in the presence of free backward or two-way classical communication, continuity was proven on the interior of the set of non-zero capacity channels.
References
[1] C. H. Bennett, D. P. DiVincenzo, and J. A. Smolin, Phys. Rev. Lett. 78, 3217 (1997)
[2] H. Barnum, A. J. Smolin, and B. Terhal, Phys. Rev. A 58, 3496 (1998)
[3] M. Keyl and R. Werner, Lecture Notes in Physics 611, 263 (2002)
[4] M. Shirokov, Comm. Math. Phys. 262, 137 (2006)
[5] M. Fannes, Comm. Math. Phys. 31, 291 (1973)
[6] M. A. Nielsen, Phys. Rev. A 61, 064301 (2000), arxiv:quant-ph/9808086
[7] M. Donald and M. Horodecki, Phys. Lett. A 264, 257 (1999), arxiv:quant-ph/9910002
[8] G. Vidal, arxiv:quant-ph/0203107
[9] R. Alicki and M. Fannes, J. Phys. A: Math. Gen. 37, L55 (2004)
[10] M. Christandl and A. Winter, J. Math. Phys. 45, 829 (2004), arxiv:quant-ph/0308088
[11] D. Leung and G. Smith, Commun. Math. Phys. 292, 201–215 (2009).